Farin [
1] used the notion of Bézier
simplices in Sibson coordinates [
2] to
propose a
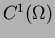
interpolant. The
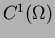
interpolant has quadratic precision,
and reduces to a cubic polynomial between adjacent nodes on
the boundary
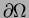
. In this
report, we present the
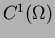
formulation and
propose a computational methodology for its
implementation in the context of a Galerkin procedure
for the solution of partial differential
equations (PDEs). The
approach involves the
transformation of the original Bernstein basis functions
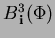
to new shape functions
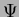
,
such that the shape functions
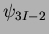
,
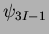
,

for node
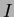
are directly
associated with the three nodal degrees of freedom
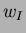
,
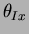
,
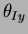
, respectively. Such a
transformation renders the interpolant
amenable to application in a Galerkin scheme for the
solution of fourth-order elliptic PDEs. Numerical
interpolation results have verified the
smoothness and quadratic precision
property of the interpolant, and currently its application to
the biharmonic equation is in progress.